Question

1.the table and the graph represent distance traveled by two cars by two different remote-controlled cars.Are both cars traveling at the same speed? If not graph a line to represent the table.t. 0 0.5 1 1.5d 0 4 8 122.write an equation for the table in problem 1.d=_t3.write an equation for the original line on the grid?4.What is the slope of the line represented in the table?5.what is the slope of the original line on the grid?5.what is the slope

Answer 1

1. No, the cars are traveling at a different sped, because the data in the table is different to the data in the graph.

The graph that represent the table is:

2. To write an equation for a line you need to find the slope (m) and the y-intercept (b):

`y=mx+b`

Slope: you use two points and the next formula:

`m=(y_2-y_1)/(x_2-x_1)`

Points: ( 0.5, 4) and ( 1, 8)

`m=(8-4)/(1.-05)=(4)/(0.5)=8`

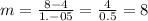
Y-intercept: Is the value of y when the line cross the y-axis (when x =0 )

Y-intercept is b=0

Then, the equation for the data in table is:
`y=8x`

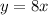
3. Use two points in the graph: ( 1, 4) and ( 2, 8)

Slope:

`m=(8-4)/(2-1)=(4)/(1)=4`

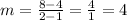
y -intercept: b=0

Then, the equation for the graph is:
`y=4x`

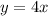
4. The slope represent the change of the distance versus the time. Then, the slope represents the speed. s= 8ft/s. The car travels 8 feet in 1 second

5. The slope represent the change of the distance versus the time. Then, the slope represents the speed. s= 4ft/s. The car travels 4 feets in 1 second.