Question

The cone has a radius of 8 feet and a height of 12 feet. What is the volume of the cone? Leave your answer in terms of π. A.32π cubic feetB. 256π cubic feetC. 374π cubic feetD. 768π cubic feet

Answer 1

The volume of the cone is 256π cubic feet. Therefore, the correct answer is B. 256π cubic feet

The formula for the volume V of a cone is given by:

V=
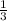`(1)/(3)` πr^2 h

where

r is the radius of the base and

h is the height of the cone.

In this case, the radius (r) is 8 feet, and the height (h) is 12 feet.

Plug these values into the formula:

V=
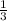`(1)/(3)` π(8^2 )⋅12

Simplify the expression:

V=
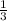`(1)/(3)` π⋅64⋅12

V=
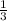`(1)/(3)` π⋅768

So, the volume of the cone is 256π cubic feet.

Therefore, the correct answer is B. 256π cubic feet

Answer 2

Given:

Cone with radius 8 feet, and height 12 feet

Recall that the formula for determining the volume of cone is

`V=(\pi r^2h)/(3)`

Given the following dimensions, substitute r = 8 ft, and h = 12 ft.

`\begin{gathered} V=(\pi r^(2)h)/(3) \\ V=\frac{\pi(8\text{ ft})^2(12\text{ ft})}{3} \\ V=\frac{(64\text{ ft}^2)(12\text{ ft})\pi}{3} \\ V=\frac{768\pi\text{ ft}^3}{3} \\ V=256\pi\text{ ft}^3 \\ \\ \text{Therefore, the volume of the cone is }256\pi\text{ ft}^3. \end{gathered}`