The circle with a radius of 6cm and a sector with a central angle measuring 60 degrees is sketched above. Note that the shaded sector that is bounded by two radii is shaded in peach color.
The area of a circle is derived as
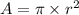
Therefore, the area of a sector (cut out of a complete 360 degree circle) is derived as a fraction of the area of a circle. The central angle of the sector will be used to determine the "fraction" of the area as shown below;

Pi is usually given as 3.14, except the question gives you another specific and different value. The solution now is;
