Question

find the x-intercept and the y-intercept for this equation 3x-5y=15a (-3,0)b (0,-3)c (0,3)d (0,5)e (5,0)

Answer 1

Given the question, the following are the solution steps to answer the question.

STEP 1: Write the given equation

`3x-5y=15`

STEP 2: Define the intercepts

The intercepts of a graph are points at which the graph crosses the axes. The x-intercept is the point at which the graph crosses the x-axis. At this point, the y-coordinate is zero. The y-intercept is the point at which the graph crosses the y-axis.

STEP 3: Find the x-intercept

To determine the x-intercept, we set y equal to zero and solve for x. This is seen below:

`\begin{gathered} 3x-5y=15 \\ set\text{ y}=0 \\ By\text{ substitution,} \\ 3x-5(0)=15 \\ 3x=15 \\ Divide\text{ both sides by 3} \\ (3x)/(3)=(15)/(3) \\ x=5 \\ \\ \therefore x-intercept=(5,0) \end{gathered}`

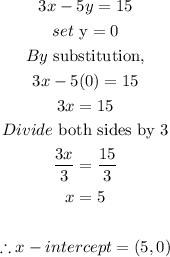
STEP 4: Find the y-intercept

Similarly, to determine the y-intercept, we set x equal to zero and solve for y. This is seen below:

`\begin{gathered} 3x-5y=15 \\ set\text{ x}=0 \\ By\text{ substitution,} \\ 3(0)-5y=15 \\ 0-5y=15 \\ -5y=15 \\ Divide\text{ both sides by -5} \\ (-5y)/(-5)=(15)/(-5) \\ y=-3 \\ \\ \therefore y-intercept=(0,-3) \end{gathered}`

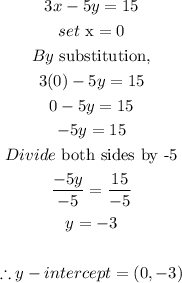
Hence,

x-intercept = (5,0)

y-intercept = (0,-3)