ANSWER

Step-by-step explanation
We want to find the factors of:
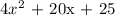
To do that we simply have to factorise the equation.
That is we need two numbers such that:
their sum = 20
their product = 4 * 25 = 100
The two numbers we need are 10 and 10.
That is:
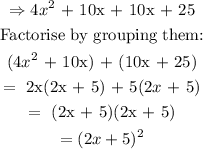
That is the answer.