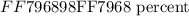Question

What percent of $658.23 is $645.07?

Answer 1

The percentage of ( a% ) is to be applied on the principal amount of ( P ):

`P\text{ = \$658.23}`

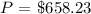
We need to determine what percentage proportion rate ( a% ) will left us with a disposable amount of ( D ):

`D\text{ = \$645.07}`

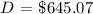
The percentage formula applied at principal amount is:

`D\text{ = P }\cdot\text{ }(a)/(100)\text{ }`

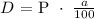
We will plug in the values the respective values as follows:

`\begin{gathered} a\text{ = }(100\cdot D)/(P) \\ a\text{ = }(100\cdot645.07)/(658.23)\text{ = }(64507)/(658.23) \\ \textcolor{#FF7968}{a}\text{\textcolor{#FF7968}{ = 98\%}} \end{gathered}`

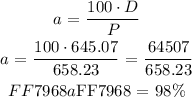
The amount of percentage reduction applied to the principal amount is ( 2% ) and $645.07 is 98% of principal $658.23.

Hence,

`\textcolor{#FF7968}{98}\text{\textcolor{#FF7968}{ percent}}`