First, we need to find the function of the line.
Use two points of the line to find the slope:
Let us choose (- 1, 0) and E ( 0 , -2) :
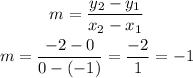
Then, the slope=m=1.
Now, we can find the equation using:
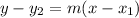
Replace using m=-2and P1(-1,0)

To find the inverse function, we need to solve for x:
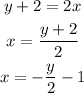
Interchange x and y:
Hence, the inverse function is:
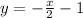
When =
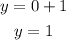
Now, we need to find two points for the graph of the inverse function.
When x=0:
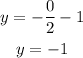
We found the point (0,-1)
When x=2
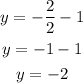
We found the point (2,-2)
Hence, the correct answers are options C and E.