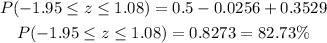a. Z-score formula

where,
• x: observed value
,
• μ: mean
,
• σ: standard deviation
Substituting with x = $275, μ = $280, and σ = $20, we get:

In terms of the z-score, we need to find
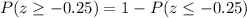
From the table:
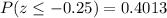
Then, the percentage of customers that has daily balances of more than $275 is:
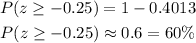
b. Substituting with x = $243, μ = $280, and σ = $20 into the z-score formula, we get:
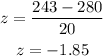
In terms of the z-score, we need to find:
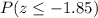
From the table, the percentage of customers that has daily balances of less than $243 is:
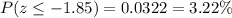
c. Substituting with x₁ = $241 and x₂ = $301.60, μ = $280, and σ = $20 into the z-score formula, we get:
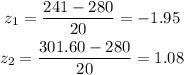
In terms of the z-score, we need to find:
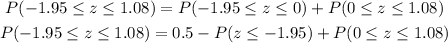
From the first table:
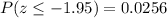
From the second table:
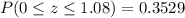
Therefore, the percentage of its customers' balances between $241 and $301.60 is: