Answer:
P(x < 3) = 0.1971
Step-by-step explanation:
To calculate the probability that x babies weigh more than 20 pounds, we will use the binomial distribution, so it can be calculated as:
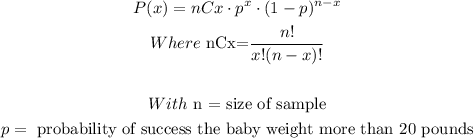
So, replacing n = 16 and p = 0.25, we get that the probability is equal to
P(x) = 16Cx (0.25)^x (1 - 0.25)^(16 - x)
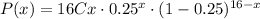
Now, the probability that fewer than three weights more than 20 pounds are equal to

Then
P(x < 3) = 0.01 + 0.0535 + 0.1336
P(x < 3) = 0.1971
Therefore, the answer is
P(x < 3) = 0.1971