Answer:
a) 0.0138
b) 0.1103
Explanations:
Probability is the likelihood or chance that an event will occur. Mathematically;
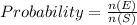
where:
n(S) is the total outcome
n(E) is the total number of events
If a well- shuffled box contains 2 gold marbles, 6 silver marbles and 9 bronze balls, the total outcome is given as:
n(S) = 2 + 6 + 9
n(S) = 17 marbles
a) If the marbles selected are two gold marbles and replaced, the probability of selecting two gold marbles will be:
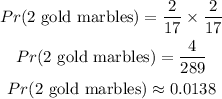
b) If two silver marbles are selected, the probability of selceting the first silver marble is given as:
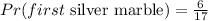
If the first silver marble is not replaced, the probability of picking the second one will be:
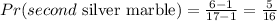
The probability that they are both silver marbles if the first marble is not replaced is calculated as:
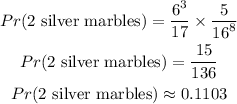
Hence the probability that they are both silver marbles if the first marble is not replaced is 0.1103