EXPLANATION
Given the function f(x) = x^2 and the point x=2
Now, we need a formula for the Distance as follows:
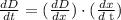
We need to compute dD/dx, this means the derivative of the distance between the points (0,0) and (2,x^2) .
The distance must be computed using the distance formula:
![D=\sqrt[]{(x-0)^2+(x^2-0)^2}](https://img.qammunity.org/2023/formulas/mathematics/college/wzj1v8v7aekegymdsdiozn3oiormdl0lbl.png)
Adding terms and computing the powers:
![D=\sqrt[]{x^2+x^4}](https://img.qammunity.org/2023/formulas/mathematics/college/7z7wtviuocn1qys0igix2k7cxz5llx5hph.png)
Rearranging terms:
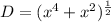
Then, we must apply the first derivative of this expression by applying the chain rule as follows:
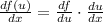
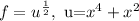
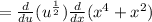
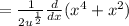
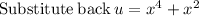
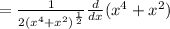
Applying the derivatives:
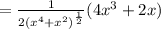
Simplifying:
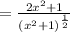
Plugging in x=2 into the expression:
![\frac{dD}{d\text{x}}_((2))=\frac{2*2^2+1}{(2^2+1)^{(1)/(2)}}=\frac{2*4+1}{(4+1)^{(1)/(2)}}=\frac{8+1}{(5)^{(1)/(2)}}=\frac{9}{\sqrt[]{5}}](https://img.qammunity.org/2023/formulas/mathematics/college/29bd63dmsuip8mf3tcpafyvuhj8j1yphwh.png)
Finally, as we already know that dx/dt = 7, we must replace terms in the expression:
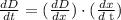
![\frac{dD}{d\text{ t}}=\frac{9}{\sqrt[]{5}}\cdot7](https://img.qammunity.org/2023/formulas/mathematics/college/7ks5zqp3nhvb9qmflanj2zgsatxoucrplx.png)
Multiplying numbers:
![\frac{dD}{d\text{ t}}=\frac{63}{\sqrt[]{5}}=\frac{63\sqrt[]{5}}{5}=28.17](https://img.qammunity.org/2023/formulas/mathematics/college/ef6fevrsmptalggiqf3cmcoqgkwj14p22q.png)
The solution is 28.17