
this is the correct way to solve these types of exercises
then we will try to do the same where a is (6+9x) and b is 24
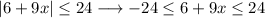
and solve the right inequality for x
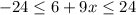
subtract 6 for all expression
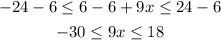
now divide on 9

ok, this means that the x values will be from -10/3 to 2 including these
Graph
solution is the red zone