Question

Match the equation y=(2x+5)(x−1) with the figure that shows the zeros, vertex, and parabola graphed correctly for the equation.

Answer 1

The given equation is:

`y=(2x+5)(x-1)`

We need to transform it into the form:

`y=ax^2+bx+c`

We can do it by multiplying the parentheses:

`\begin{gathered} y=2x\cdot x-2x\cdot1+5\cdot x-5\cdot1 \\ y=2x^2-2x+5x-5 \\ y=2x^2+3x-5 \end{gathered}`

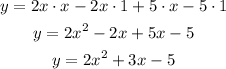
Then a=2, b=3 and c=-5.

The vertex of the parabola is given by (h,k) where:

`\begin{gathered} h=-(b)/(2a) \\ k=f(h) \end{gathered}`

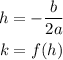
Then h and k are equal to:

`\begin{gathered} h=-(3)/(2\cdot2)=-(3)/(4)=-0.75 \\ k=f(-0.75) \\ \therefore k=2\cdot(-0.75)^2+3(-0.5)-5 \\ k=1.125-2.25-5 \\ k=-6.13 \end{gathered}`

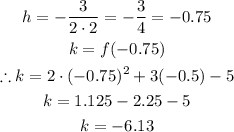
Thus, the vertex is at (-0.75, -6.13).

Now, to find the zeros of the function, we can use the quadratic formula:

`x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}`

By replacing the know values we obtain:

`\begin{gathered} x=\frac{-3\pm\sqrt[]{3^2-4(2)(-5)}}{2(2)} \\ x=\frac{-3\pm\sqrt[]{9+40}}{4} \\ x=\frac{-3\pm\sqrt[]{49}}{4} \\ x=(-3\pm7)/(4) \\ \text{Then x is equal to:} \\ x=(-3+7)/(4)=(4)/(4)=1\text{ and }x=(-3-7)/(4)=(-10)/(4)=-2.5 \end{gathered}`

Thus, the zeros are at: (-2.5,0) and (1,0).

Answer: the graph that represents the parabola is the last one with the vertex at (-0.75, -6.13) and the zeros at (-2.5,0) and (1,0).