Question

Write the equation of the line that is parallel to the line 2x + 3y = 5 and contains the point (-2,1). O A 2x + 3y = -1 O B. 3x + 2y = 5 O c. 3x-2y=7 O D. 2x - 3y=7

Answer 1

Step 1

The equation of a line is given as

`\begin{gathered} y=mx+c \\ \text{where } \\ m\text{ = slope} \\ c\text{ = y-intercept} \end{gathered}`

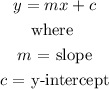
The given equation is

`\begin{gathered} 2x+3y\text{ = 5} \\ 3y=5-2x \\ y=(5)/(3)-(2x)/(3) \\ m=-(2)/(3) \end{gathered}`

Step 2

For parallel lines their slopes are equal

`\text{Hence the slope of the line parallel to the given equation = -}(2)/(3)`

The equation of the required parallel line now becomes

`y=c-(2)/(3)x`

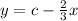
Step 3

Find the y-intercept

`\begin{gathered} \text{The given points are ( -2,1) in the form of (x,y)} \\ \text{Hence,} \\ 1=c-(2)/(3)(-2) \\ 1-(4)/(3)=c \\ c=-(1)/(3) \end{gathered}`

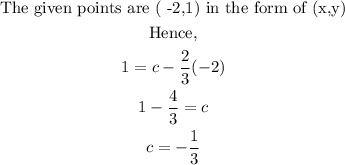
Step 4

Write the required equation

`\begin{gathered} y=-(1)/(3)-(2)/(3)x \\ 3y=-1-2x \\ 2x+3y=-1 \end{gathered}`

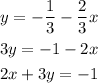
The answer is 2x+3y = -1