Given the equation:
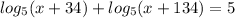
Apply the product property of logarithm.
Since they have the same bases, we have:
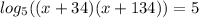
Now, expand using FOIL method and apply distributive property:
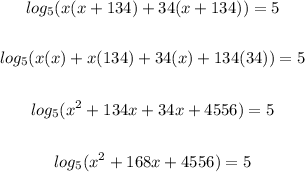
Rewrite the equation using the definition of logarithm:
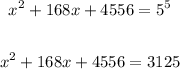
Equate to zero.
Subtract 3125 from both sides:
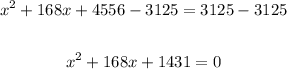
Factor the left side of the equation using AC method.
Find a pair of numbers whose sum is 168 and product is 1431
We have:
9 and 159
We nor have the factored form:
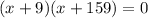
Equate each factor to zero and solve for x:

Hence, we have the solutions:
x = -9 and -159
To find the real solution substitute each value of x in the equation to determine which solution makes the equation true.
When x = -9:
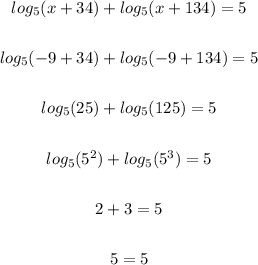
-9 makes the logarithm equation true.
When x = -159:
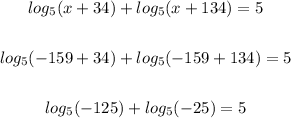
Since we have the log of negative numbers, the equation is undefined when x = -159.
Therefore, the correct solution is x = -9.
The solution set is {-9}
ANSWER:
A. The solution set is {-9}