Given the equation:
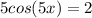
Let's solve the equation for the smallest three positive solutions.
The first step is to divide both sides of the equation by 5:
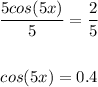
Take the cos inverse of both sides:
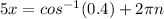
Where n is any integer.
Now, divide both sides by 5:
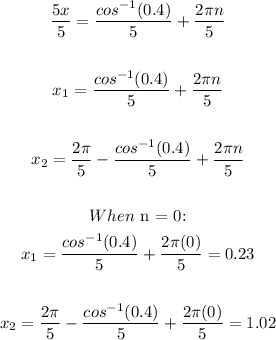
When n = 1:
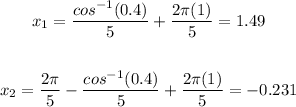
When n = 2:
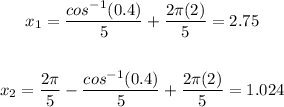
Therefore, the smallest three positive solutions are:
x = 0.23, 1.02, 1.49
ANSWER:
0.23, 1.02, 1.49