Question

Which of the following is not the property of the absolute value function f(x) = |x|?An absolute value function is an odd function.The domain of f(x) =|x| is the set of all real numbers.The range is the set of real numbers greater than or equal to zero.It is strictly increasing in [0, ∞) and strictly decreasing in (-∞, 0].

Answer 1

Given:

The given function is f(x)=|x|.

Required:

We need to find the property of the given function.

Explanation:

Consider the graph of the function.

The domain of a graph consists of all the input values shown on the x-axis.

The domain of f(x) =|x| is the set of all real numbers.

The range of a graph consists of all the output values shown on the y-axis.

The range is the set of real numbers greater than or equal to zero.

It is strictly increasing in [0, ∞) and strictly decreasing in (-∞, 0] is true.

A function is odd if −f(x) = f(−x), for all x

`f(-x)=|-x|=|x|=f(x)`

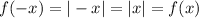
The absolute value function is not an odd function.

Final answer:

The wrong property is

The absolute value function is an odd function.