Question

3. Which value is a possible solution to thefollowing inequality?4 - 3x > -14A. 7B. 10C. 8D. 5

Answer 1

the expression we have is:

`4-3x>-14`

To solve this, we substract 4 from both sides of the inequality:

`\begin{gathered} 4-4-3x>-14-4 \\ -3x>-18 \end{gathered}`

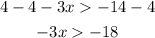
Next, we divide both sides by (-3), but we have to flip the symbol > for a <, this is because when we divide by a negative number we have to flip the symbol.

`(-3x)/(-3)<(-18)/(-3)`

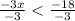
solving the operations:

`x<6`

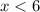
And the only one of our options that is less than 6 is option D.5