Question

You need a 75% alcohol solution. On hand, you have a 25mL of a 5% alcohol mixture. You also have 80% alcohol mixture. How much of the 80% mixture will you need to ADD to obtain the desired solution?

Answer 1

It is given that 75% alcohol is needed. It is also given that 25mL of a 5% alcohol mixture and 80% alcohol mixture is owned.

It is required to find the amount of 80% mixture that will be needed to obtain the desired solution.

Let x be the amount of 80% mixture.

Since I have 25mL of 50% and 75% of the solution is needed, the required equation is:

`80\%x+5\%(25)=75\%(x+25)`

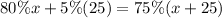
Solve the resulting equation for x:

`\begin{gathered} (80)/(100)x+(5)/(100)(25)=(75)/(100)(x+25) \\ \Rightarrow(80)/(100)x+(5)/(100)(25)=(75)/(100)x+(75)/(100)(25) \\ \Rightarrow(4)/(5)x+(5)/(4)=(3)/(4)x+(75)/(4) \\ \Rightarrow(4)/(5)x-(3)/(4)x=(75)/(4)-(5)/(4) \\ \Rightarrow(1)/(20)x=(35)/(2) \\ \Rightarrow2x=20*35 \\ \Rightarrow(2x)/(2)=(20*35)/(2) \\ \Rightarrow x=10*35 \\ \Rightarrow x=350 \end{gathered}`

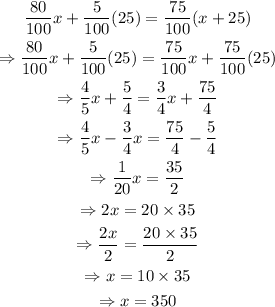
Hence, you'll need 350mL of the 80% mixture to get a 75% alcohol solution.

The answer is 350mL.