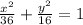Given:
The vertices of the ellipse are at (0,6) and (0,-6) and co-vertices at (4,0) and (-4,0).
Required:
We have to find the equation of the ellipse.
Step-by-step explanation:
The vertices are at (0,6) and (0,-6) then the length of the major axis is 6.
The co-vertices are at (4,0) and (-4,0) then the length of the minor axis is 4.
We know that the standard form of an ellipse is
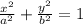
Where a and b are the length of the major axis and the minor axis respectively.
Then the required equation of the ellipse is
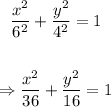
Final answer:
Hence the final answer is