Question

a countertop is in the shape of a trapezoid. the lengths of the bases are 70 1 over 2. and 65 1 over 2 inches long. the area of the countertop is 1225 square inches. write and solve an equation to find the height of the countertop

Answer 1

Let's begin by identifying key information given to us:

The figure is a trapezoid

base (1) = 70 1/2 in = 70.5 in

base (2) = 65 1/2 in = 65.5 in

area = 1224 in sq

To solve for the height, we will follow these steps:

`\begin{gathered} A=((a+b)/(2))h \\ A=1224in^2,a=70(1)/(2)in\text{ or 70.5 in},b=65(1)/(2)in\text{ or 65.5 in},h=\text{?} \\ 1224=(\mleft(70.5+65.5\mright))/(2)_{}h\Rightarrow1224=(136)/(2)h \\ 1224\cdot2=136h\Rightarrow136h=2448 \\ \Rightarrow h=(2448)/(136)=18 \\ h=18in \\ \\ \therefore The\text{ height of the countertop is }18in \end{gathered}`

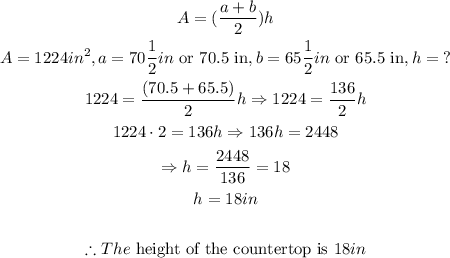
Therefore, the height of the countertop is 18 inches