Ok, so
Here we have the following function:
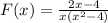
We want to check if the discontinuity of F(x) is removable or non removable.
We could factor the expression in the denominator using the following property:
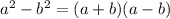
And we could apply the common factor property in the numerator.
Then,
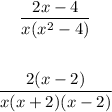
We can cancel the "x-2" term.
In the above expression due to (x-2) in the denominator, f(x) becomes undefined at x=2, Hence there is a discontinuity at x=2. However this (x-2) gets cancelled by (x-2) in the numerator, and we obtain:

Notice that the new expression has a discontinuity at the points x=0 and x=-2. For this reason, there is a non removable discontinuity at x=0 and x=-2, because the function becomes undefined at these points and there's not a way to cancel them.