Question

On a unit circle, the terminal point of theta is (1/2,square root 3/2). what is theta

Answer 1

Given the terminal point of Θ:

`((1)/(2),\frac{\sqrt[]{3}}{2})`

Let's find the value of Θ.

In polar coordinates, we have the points as

`P=(R,\theta)`

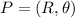
Where:

R is the radius and Θ is the angle.

We know in rectangular coordinates, we have:

x = R * cosΘ

Y = R * sinΘ

Thus, to find the value of Θ, we have:

`\sin \theta=\frac{\sqrt[]{3}}{2}`

Solve for Θ.

`\begin{gathered} \\ \text{sin}\theta=\frac{\sqrt[]{3}}{2} \\ \end{gathered}`

Take the inverse cosine of both sides:

`\begin{gathered} \theta=\sin ^(-1)(\frac{\sqrt[]{3}}{2}) \\ \\ \theta=(\pi)/(3) \end{gathered}`

ANSWER:

`C.\text{ }(\pi)/(3)radians`