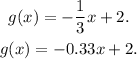Recall that two lines are perpendicular if the product of their slopes is equal to -1.
Notice that f(x) is given in slope-intercept form, then, the slope of f(x) is 3. Therefore, the slope of g(x) must be
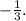
Now, to determine the equation of g(x) we will use the following formula:
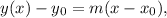
where (x₀,y₀) is a point on the line, and m is the slope.
Substituting m=-1/3 and (x₀,y₀)=(3,1), we get:
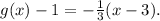
Taking the above equation to its slope-intercept form we get:
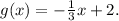
Answer:
Slope:

Equation: