Question

Anna Litical is riding on The Shock Wave at Great America. Anna experiences adownward acceleration of 12.5 m/s2 at the top of the loop and an upward acceleration of24.0 m/s2 at the bottom of the loop. Use Newton's second law to determine the normalforce acting upon Anna's 50-kg body at the top and at the bottom of the loop.

Answer 1

The normal force at the top is 135N and the normal force at the bottom is 1690 N.

Step-by-step explanation:

At the top and the bottom of the loop, we have the following free body diagrams

Then, for the top of the loop and by the second law of newton, we can write the following equation

`\begin{gathered} F_(net)=F_n+mg=ma \\ F_n=ma-mg \end{gathered}`

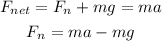
Where m is the mass, a is the downward acceleration, and g is the gravity. Replacing m = 50 kg, a = 12.5 m/s², and g = 9.8 m/s², we get that the normal force at the top of the loop is

`\begin{gathered} F_n=(50\text{ kg\rparen\lparen12.5 m/s}^2)-(50\text{ kg\rparen\lparen9.8 m/s}^2) \\ F_n=625N-490N \\ F_n=135\text{ N} \end{gathered}`

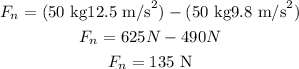
In the same way, we can write the following equation for the bottom of the loop

`\begin{gathered} F_(net)=F_n-mg=ma \\ F_n=ma+mg \end{gathered}`

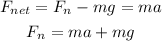
Replacing m = 50 kg, a = 24.0 m/s², and g = 9.8 m/s², we get that the normal force at the bottom of the loop is

`\begin{gathered} F_n=(50\text{ kg\rparen\lparen24.0 m/s}^2)+(50\text{ kg\rparen\lparen9.8 m/s}^2) \\ F_n=1200\text{ N + 490 N} \\ F_n=1690\text{ N} \end{gathered}`

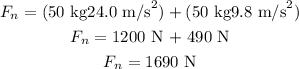
Therefore, the normal force at the top is 135N and the normal force at the bottom is 1690 N.