Question

DetailsA farmer has a 100 ft by 50 ft rectangular field that he wants to increase by 25.28% by cultivating a strip ofuniform width around the current field. How wide of a strip should he cultivate around the edge of his fieldto do this?

Answer 1

The wide of a strip he should cultivate is 4ft

Given

A farmer has a 100 ft by 50 ft rectangular field that he wants to increase by 25.28% by cultivating a strip of uniform width around the current field

Solution

Since, the increment is by 25.28%

`((100+2w)(50+2w)-100(50))/(100(50))=0.2528`

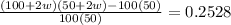
We cross multiply and solve for w

We can now solve the quadratic equation by factorisation

`\begin{gathered} w^2-4w+79w-316=0 \\ (w^2-4w)+(79w-316)=0 \\ w(w^{}-4)+79(w-4)=0 \\ (w-4)\text{ (w+79)=0} \\ w=4 \\ w=-79 \\ \end{gathered}`

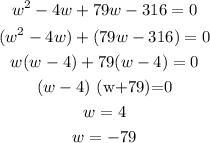
Distance can't be negative

Therefore, the wide of a strip he should cultivate is 4ft