Write a verbal description for each step of the equation solving process used to solve the following equations for x.

Question

asked Nov 9, 2023 43.9k views

1 Answer

Matti Mehtonen · Answer 1 · 2023-11-15T18:01:47+0000

Okay, here we have this equation:

$r\cdot\sqrt[]{(mx)/(n)+s}=t$

And we need write the verbal description to solve for x, let's do it:

We first move on to dividing the "r" on the right side of the equation, the equation remains like this for now:

$\sqrt[]{(mx)/(n)+s}=(t)/(r)$

The second step is to square both sides to cancel the radical on the left, the equation remains like this for now:

$\begin{gathered} \sqrt[]{(mx)/(n)+s}^2=((t)/(r))^2 \\ (mx)/(n)+s=((t)/(r))^2 \end{gathered}$

The third step is to go on to subtract the s from the right side, and the equation looks like this:

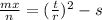
The fourth step is to multiply the "n" on the right side of the equation:

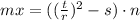
$mx=(((t)/(r))^2-s)\cdot n$

And the last step is to divide the "m" on the right side of the equation, and we finally get this:

$x=(((((t)/(r))^2-s)\cdot n))/(m)$