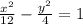Answer:
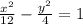
Explanation:
Since our foci are located on the x-axis, then our major axis is going to be the horizontal transverse axis of the hyperbola:
Formula for hyperbola with horizontal transverse axis centered at origin
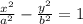
- Directrices ->

- Foci ->
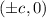 where
where

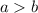
Since we are given our directrices of
 and foci of
and foci of
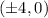 , then we can set up the directrices equation to solve for
, then we can set up the directrices equation to solve for
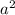 :
:

Now we can determine
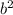 to complete our equation for the hyperbola:
to complete our equation for the hyperbola:
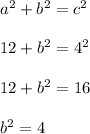
Therefore, our equation for our hyperbola is