Given:
• YM = 15
,
• YN = 8
,
• LN = 10
Let's solve for the missing side lengths and angles.
We have the following:
• Measure of angle NLY:
Since traingle NLY is a right traingle, to find the measure of angle NLY, apply trigonometric ratio.
Here, let's apply the trigonometric ratio formula for sine:
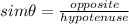
Where:
θ is the angle
opposite side is the side opposite the angle = YN = 8
Hypotneuse = LN = 10
Thus, we have:
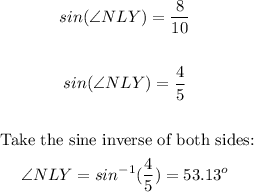
Therefore, m∠NLY = 53.13°.
• Measure of angle NMY:
Apply the trigonometric ratio formula for tangent:

Where:
θ is the angle
The opposite side is the side opposite the angle = YN = 8
Adjacent side is the side adjacent the angle = YM = 15.
Thus, we have:
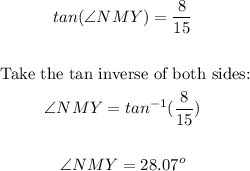
Therefore, m∠NMY = 28.07°.
• Length of NM:
To find the length of NM, apply Pythagorean Theorem:
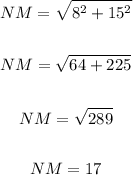
The length of NM is 17.
• Length of LY:
To find the length of LY, also apply Pythagorean theorem:
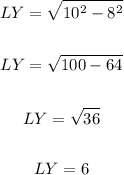
ANSWER:
• m∠NLY ==> 53.13°
,
• m∠NMY ==> 28.07°
,
• NM ==> 17
,
• LY ==> 6