Question

There are 2 questions I need help with. I uploaded them both in a picture. Thank you

Answer 1

Given:

Pure alcohol is added to 180 gallons of 7% alcohol solution to produce a solution that is 10%

To find:

Amount of pure alcohol.

Step-by-step explanation:

Let

x = number of gallons of 100% alcohol solution

180 = number of gallons of 7% alcohol solution

x + 180 = number of gallons of 10% alcohol solution

The amount of alcohol in a solution is found by multiplying the number of gallons of solution by the percentage (as a decimal) of alcohol that the solution contains.

Alcohol content of 100% solution

`1* x=x`

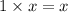
Alcohol content of 7% solution is:

`0.07*180=12.6`

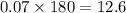
Alcohol content of mixture (10% solution) is:

`\begin{gathered} 0.1*(x+180) \\ =0.1x+18 \end{gathered}`

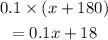
Now,

`\begin{gathered} x+12.6=0.1x+18 \\ \Rightarrow x-0.1x=18-12.6 \\ \Rightarrow0.9x=5.4 \\ \Rightarrow x=(5.4)/(0.9) \\ \Rightarrow x=6\text{ gallons} \end{gathered}`

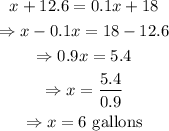
Final answer:

Hence, the required solution is 6 gallons.