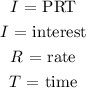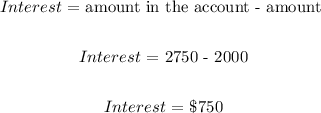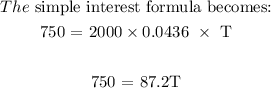Question

Suppose you have $2750 in your savings account at the end of a certain period of time. You invested $2000 at a 4.36% simple annual interest rate. How long, in years, was your money invested ? (State your results to the nearest hundredth of a year.)

Answer 1

Answer:

The money was invested for 8.60 years

Step-by-step explanation:

Given:

Amount in the savings account = $2750

Amount invested = $2000

rate = 4.36% = 0.0436

To find:

the time it took to invest the money to obtain the amount in the account

The investment was done using simple interest. So to get the time, we will apply simple interest formula

`\begin{gathered} I\text{ = PRT} \\ I\text{ = interest} \\ R\text{ = rate} \\ T\text{ = time} \end{gathered}`
`\begin{gathered} Interest\text{ = amount in the account - amount } \\ \\ Interest\text{ = 2750 - 2000} \\ \\ Interest\text{ = \$750} \end{gathered}`
`\begin{gathered} The\text{ simple interest formula becomes:} \\ 750\text{ = 2000}*0.0436\text{ }*\text{ T} \\ \\ 750\text{ = 87.2T} \end{gathered}`
`\begin{gathered} divide\text{ both sides by 87.2:} \\ T\text{ = }(750)/(87.2) \\ \\ T\text{ = 8.60 year} \end{gathered}`