31.
The domain of a function is the set of all values that its variable can take.
To find the domain of a function, search for restrictions over its variable in its rule of correspondence. Remember that common restrictions are:
1. The denominator must be different to 0.
2. The argument of square roots cannot be negative.
For the given function:

In this rule of correspondence, the variable x does not appear in a denominator or inside a square root. Then, there are no restrictions over the variable x and the domain is the set of all real numbers, which can be represented using interval notation as:
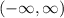
To sketch the graph of the function, notice that it is the equation of a line with slope -1 and y-intercept 4: