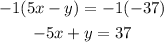Given the points;
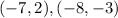
The slope-intercept form of the equation of the line that passes through the pair of points is;
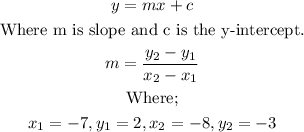
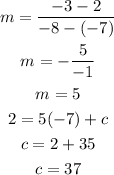
Thus, the slope-intercept form of the equation of the line is;
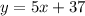
Then, the equation in the form of Ax+By = C is;
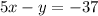
Or we multiply through by -1, we have;