Question

Using pythagorean theorem determine the following lengths make up a right triangle. 8cm, 6cm, 9cm

Answer 1

We have the following lengths for the triangle, which we will label as a, b and c:

To check if they form a right triangle, we have to use the Pythagorean Theorem:

`a^2+b^2=c^2`

If we substitute the values and get the same result on both sides, the Pythagorean theorem condition will be met and the three sides will form a right triangle.

Substituting a, b, and c into the Pythagorean Theorem:

`8^2+6^2=9^2`

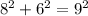
Solving the squared terms on the left and right sides of the equation:

`64+36=81`

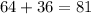
Adding 64+36 on the left side, we get:

`100=81`

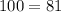
As we can see, we get 100 on the left side and 81 on the right side, since 100 is not equal to 81, The three lengths don't form a right triangle.

Answer: The lengths 8cm, 6cm, and 9cm don't make up a right triangle.