Question

A large waffle cone at yummy ice cream is 6 inches tall and has a radius of 3 inches. The small waffle cone is 3 inches high and has a radius of 1 inch.How much more ice cream will the large cone hold that the small cone?

Answer 1

The large cone will hold 53.41 cubic inches more of ice cream.

Step-by-step explanation:

Since the problem deals with how much ice cream the cones will hold, we are to calculate volumes for the small and large cones.

`\text{Volume of a cone=}(1)/(3)\pi r^2h`

Large Cone

Height = 6 inches

Radius = 3 inches

`\begin{gathered} \text{Volume}=(1)/(3)*\pi*3^2*6 \\ =18\pi\; in^2 \end{gathered}`

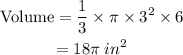
Small Cone

Height = 3 inches

Radius = 1 inches

`\begin{gathered} \text{Volume}=(1)/(3)*\pi*1^2*3 \\ =\pi\; in^2 \end{gathered}`

Therefore, the difference in volume

`\begin{gathered} =18\pi-\pi \\ =17\pi \\ =53.41\; in^2 \end{gathered}`

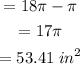
The large cone will hold 53.41 cubic inches more of ice cream.