EXPLANATION
Given the sequence: -71, -85.2, -102.24, -122.688
The common ratio is given by the expression:
a_n = a_0* r^(n-1)
Check wheter the ratio is constant:
-71, -85.2, -102.24, -122.688
Compute the ratios of all the adjacent terms:
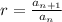
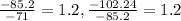
The ratio of all the adjacent terms is the same and equl to
r=1.2
The first element of the sequence is:
a_1 = -71