SOLUTION
From the question,
The cost is given by the function
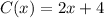
And the price demand function is given by the function
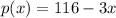
Now profit is calculated as
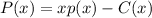
So we have
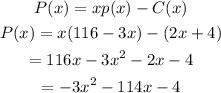
Hence the profit function is
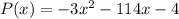
At maximum profit, the derivative of the function for profit is equal to zero, we have
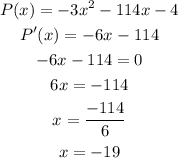
So we can see that the answer to that is -19
The maximum profit becomes ,
we substitute x for -19, we have
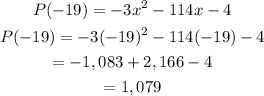
Hence the maximum profit is $1,079