Question

Kyle starts at rest at the top of a 9m ramp 30°on his skateboard, How fast is he traveling atbottom?

Answer 1

Given,

The initial velocity of Kyle, u=0 m/s

The length of the ramp, d=9 m

The angle of inclination of the ramp, θ=30°

The acceleration of the skateboard and Kyle is given by,

`a=g\sin \theta`

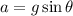
Where g is the acceleration due to gravity.

On substituting the know values,

`\begin{gathered} a=9.8*\sin 30^(\circ) \\ =4.9m/s^2 \end{gathered}`

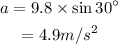
From the equation of motion, the velocity of Kyle and the skateboard at the bottom of the ramp is given by,

`v^2=u^2+2ad`

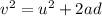
On substituting the known values,

`\begin{gathered} v^2=0+2*4.9*9 \\ v=\sqrt[]{88.2} \\ =9.39\text{ m/s} \end{gathered}`

Thus Kyle is traveling with a velocity of 9.39 m/s at the bottom of the ramp.