Question

11.A rock is thrown down with an initial speed of30.0 ft/s from a bridge to the water below. It takes3.50 s for the rock to hit the water.Find the speed (in ft/s) of the rock as it hitsthe water.a.b.How high is the bridge above the water?

Answer 1

a)

The final speed v_f can be calculated from the formula for the acceleration:

`v_f=v_0+gt`

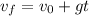
Replace v_0=30.0ft/s, g=32.2ft/s^2 and t=3.50s to find the final speed of the rock:

`v_f=30.0(ft)/(s)+(32.2(ft)/(s^2))(3.50s)=142.7(ft)/(s)`

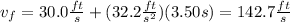
Therefore, the speed of the rock as it hits the water is approximately 143 ft/s.

b)

The distance d traveled by an object during a time interval t is given by the average speed as follows:

`d=\bar{v}\cdot t`

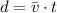
On the other hand, an object in free fall accelerates uniformly. The average speed of an object under uniformly accelerated motion is:

`\bar{v}=\frac{v_f+v_0_{}}{2}`

Then, for uniformly accelerated motion, the distance traveled by an object that changes its velocity from v_0 to v_f during a time interval t, is:

`d=(v_f+v_0)/(2)\cdot t`

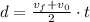
Notice that the initial speed of the rock is known, as well as the time interval t during which the rock falls. The final speed was calculated in part (a). Then, the distance that the rock travels during the time interval of 3.50s is:

`d=(142.7(ft)/(s)+30.0(ft)/(s))/(2)*3.50s=302.225ft`

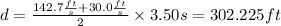
Therefore, the height of the bridge above the water is approximately 302 feet.