Given:
Let x be the principal.
Betty invested some money at 11 % interest.
That is,
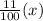
Betty also invested $240 more than 5 times that amount at 13%.
That is,

Since, Betty receives $1344.48 in interest after one year
Therefore,
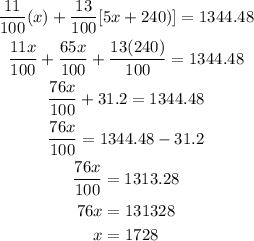
Hence, the investment at 11% is $1728.
The investment at 13% is,
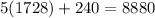
The investment at 13% is, $8880.