Question

A native wolf species has been reintroduced into a national forest. Originally 200 wolves were transplanted. After 3 years, the population had grown to 270 wolves. If the population grows exponentially, then at what annual rate is the population growing? Round the answer to the nearest tenth of a percent.

Answer 1

The population is growing at an annual rate of 16.2%

Here, we want to calculate the exponential growth rate

Mathematically, we can write the exponential equation of growth as follows;

`\begin{gathered} P=I(1+r)^t \\ \\ \end{gathered}`

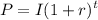
Where P is the population after a certain number of years ( 270 after 3 years

I is the initial popultaion which is 200

r is the percentage we want to calculate

t is the number of yeats to reach P which is 3 in this case

`\begin{gathered} 270=200(1+r)^3 \\ (1+r)^{3\text{ }}\text{ = }(270)/(200) \\ \\ (1+r)^3\text{ = 1.35} \\ \\ 1\text{ + r = }\sqrt[3]{1.35} \\ \\ 1\text{ + r = 1.162} \\ \\ r\text{ = 1.162 - 1} \\ \\ r\text{ = 0.162} \end{gathered}`

To the nearest tenth of a percentage, this is 16.2%