we have
20 objects
8 black
7 red
rest white
20-8-7= 5
5 white
since we are selecting 3 object at once
1) probability that one is black, one is red and the remaining one is white.
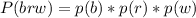
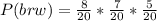
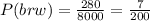

1) probability one is black, one is red and the remaining one is white. is 0.035 or 3,5%
ii) exactly two are red.
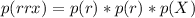
lets assume x is any ball
then p(x) is the probability of ge any ball = 1
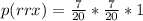
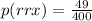
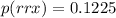
the probability of exactly two are red. is 0.1225 or 12,25%
iii)none of them is white.

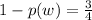
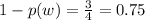
the probability that none is white is 0.75 or 75%