Question

Which of the following would best be solved using factoring the difference of squares? 2² + 3x - 10 = 0 O 3r² + 12x = 8 2³ +52² - 9x - 45 = 0 2²-25=0

Answer 1

Given: Different equations

To Determine: Which would be best solved using difference of two squares

Solution

The factorization of a difference of two squares is given below

`a^2-b^2=(a-b)(a+b)`

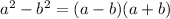
Let us examine each of the given equation

`\begin{gathered} x^2+3x-10=0 \\ x^2-2x+5x-10=0 \\ x(x-2)+5(x-2)=0 \\ (x-2)(x+5)=0 \end{gathered}`
`\begin{gathered} 3x^2+12x=8 \\ 3x^2+12x-8=0 \end{gathered}`
`\begin{gathered} x^3+5x^2-9x-45=0 \\ x^2(x+5)-9(x+5)=0 \\ (x+5)(x^2-9)=0 \\ x^2-9=x^2-3^2=(x+3)(x-3) \\ Therefore \\ (x+5)(x+3)(x-3)=0 \end{gathered}`
`\begin{gathered} x^2-25=0 \\ x^2-5^2=0 \\ (x+5)(x-5)=0 \end{gathered}`

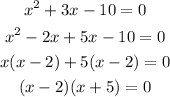
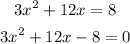
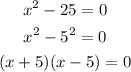
From the above,

`\begin{gathered} x^3+5x^2-9x-45=0 \\ AND \\ x^2-25=0 \end{gathered}`

The two equation above can be solved by difference of two square, but the equation below is the easiest solved using differnce of two square

x² - 25 = 0