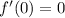Question

Find the instantaneous rate of change of the function at the given value . Thank you

Answer 1

The instantaneous rate of change of the function at x=0 is 0

`f^(\prime)(0)=0`

Step-by-step explanation:

We want to find the instantaneous rate of change of the function below;

`y=2x^2-2`

at x =0.

The instanteneous rate of change of function f(x) at point a can be written as;

`f^(\prime)(a)=(df(a))/(dx)`

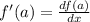
For the given function;

`f^(\prime)(x)=y^(\prime)=4x`

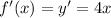
so, at x=0;

`\begin{gathered} f^(\prime)(x)=4x \\ f^(\prime)(0)=4(0) \\ f^(\prime)(0)=0 \end{gathered}`

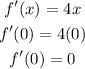
Therefore, the instantaneous rate of change of the function at x=0 is 0

`f^(\prime)(0)=0`