Solution
- In order to find the shapes that will balance out scale C, we need to analyze scales A and B.
- Let the weight of a cylinder be C, the weight of a sphere be S, and the weight of a cube be B.
On Scale A:
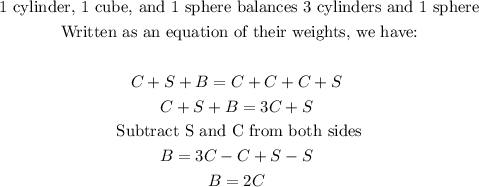
- This implies that 2 cylinders weigh just as much as 1 Cube.
On Scale B:
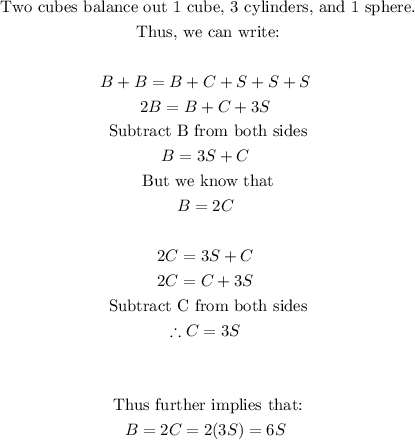
- This implies that 3 spheres weigh as much as 1 cylinder and 6 spheres weigh as much as 1 Cube.
On Scale C:

Final Answer
The answer is 1 CYLINDER on the left platform of Scale C