Answer:
P = 0.273
Step-by-step explanation:
The probability to get exactly 4 heads can be calculated using the binomial distribution because we have n identical events with a probability p of success. So, we can use the following equation:
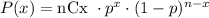
Where n is the number of times that the coin is tossed, x is the number of heads and p is the probability to land heads.
Additionally, nCx is calculated as:
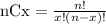
So, replacing n by 8, x by 4, and p by 0.5, we get:
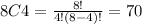
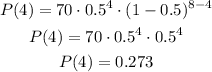
Therefore, the probability that the coin will land heads 4 times is 0.273