f°g(x) = f(g(x))
To fid it we need to replace x = g(x) into f(x) as follows:

Since the obtained function is a polynomial, its domain and range are all real numbers.
g°f(x) = g(f(x))
To fid it we need to replace x = f(x) into g(x) as follows:
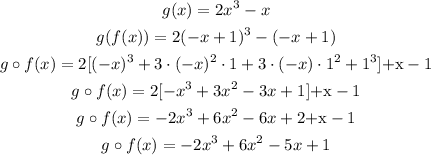
Since the obtained function is a polynomial, its domain and range are all real numbers.