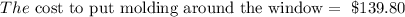Question

Need the answer no is able to give me the right answer

Answer 1

Given:

The window is divided into a semi-circle and a rectangle.

The radius of the semi-circle is 2' 9''.

The length of the rectangle is 5; 6''.

The cost of put molding for the curved portion is $10.82 per foot.

The cost of put molding for the straight portion is $ 2.81 per foot.

Required:

We need to find the cost to put molding for the window.

Step-by-step explanation:

Convert inches to feet.

`We\text{ know that 1 foot =12 inches.}`

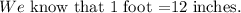
Divide 9 inches by 12 to convert inches to feet.

`9\text{ inches=}(9)/(12)feet=(3)/(4)feet.`
`2^(\prime)9^(\prime)^(\prime)=2+(3)/(4)=(8+3)/(4)=(11)/(4)feet.`
`The\text{ radius of the semi-circle, r =}(11)/(4)feet.`

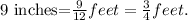
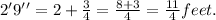
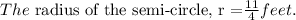
Divide 6 inches by 12 to convert inches to feet.

`6\text{ inches=}(6)/(12)feet=(1)/(2)feet.`
`The\text{ length of the rectangle is, }l=5+(1)/(2)feet=(11)/(2)feet`

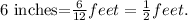
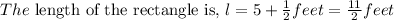
The width of the rectangle is the same as the diameter of the semi-circle.

`d=2r=2*(11)/(4)=(11)/(2)feet.`
`The\text{ width of the rectangle is, w=}(11)/(2)feet.`

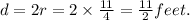
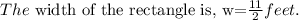
Consider the arc length of the semi-circle formula.

`S=\pi r`

`\text{ Substitute }r=(11)/(4)\text{ and }\pi=3.14\text{ in the formula.}`
`S=3.14*(11)/(4)`
`S=8.635feet.`

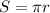
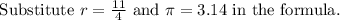
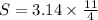
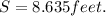
Multiply the arc length by $10.82 to find the cost of put molding for the curved portion.

`The\text{ cost of the curved portion=8.635}*10.82`
`The\text{ cost of the curved portion=\$ 93.4307.}`

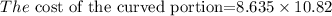
The perimeter of the straight portion is the sum of the three sides of the rectangle.

`P=l+w+l`
`Substitute\text{ }l=\frac{11}{2\text{ }}feet\text{ and }w=(11)/(2)feet\text{ in the formula.}`
`P=(11)/(2)+(11)/(2)+(11)/(2)`
`P=16.5`

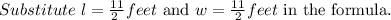
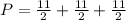
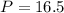
Multiply the perimeter by $2.81 to find the cost of put molding for the straight portion.

`The\text{ cost of the straight portion}=16.5*2.81`
`The\text{ cost of the straight portion}=46.365`

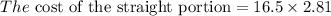
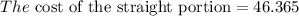
The cost to put molding around the window is the sum of the cost of the curved portion and straight portion.

`The\text{ cost to put molding around the window}=\text{ \$93.4307+ \$}46.365`
`The\text{ cost to put molding around the window}=\text{ \$139.7957}`
`The\text{ cost to put molding around the window}=\text{ \$139.80}`

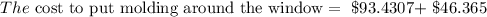
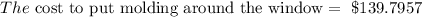
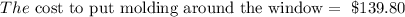
Final answer:

`The\text{ cost to put molding around the window}=\text{ \$139.80}`