Question

A. How far is k from EH? and ED?B. what.l can you conclude about EK?C. fund the value of XD. find the measure of angle DEH

Answer 1

Notice that two right triangles are formed in the diagram.

Both triangles are congruent due to the Hypotenuse-Leg theorem, since in both triangles, KE is the hypotenyse (the side opposed to the right angle).

Since both triangles are congruent, the angles opposite to equal sides must be equal. Then:

`2x=x+20`

Substract x from both sides:

`\begin{gathered} 2x-x=x+20-x \\ \Rightarrow x=20 \end{gathered}`

Then, the angle opposite to the side of length 10 has a measure of 40º, since x+20 equals 20+20, which is equal to 40.

Use the sine trigonometric function to find the length of the hypotenuse KE:

`\begin{gathered} \sin (40)=(10)/(KE) \\ \Rightarrow KE=(10)/(\sin (40)) \end{gathered}`

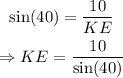
Use a calculator to find the value of 10/sin(40), setting the calculator in degrees.

`EK=15.557\ldots`

Notice that the distance from K to EH is 10, as specified by the diagram (the distance from a point to a line is equal to that of a segment perpendicular to the line).

Therefore, the answers are:

a) 10

b) EK = 10/sin(40) = 15.557..., it is the hypotenuse of a right triangle.

c) x=20.