We are given a problem that can be solved using an inequality. We are told that Toni can carry a maximum of 17 lb. Therefore, the sum of the weights inside her backpack must be smaller or equal to 17 lb. The sum of the weight inside her backpack is 1 lb lunch, 2 lb clothes, and 2n books (n = number of books). We can write this mathematically like this:
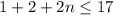
Now we solve for "n", first by solving the operations, like this:
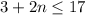
Now we subtract 3 on both sides of the inequality:
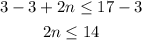
Now we divide both sides by 2
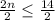

This means that Toni can carry at most 7 books